Searching for my Point of Origin
We all have a unique path that we follow through the 4 dimensions of Space and Time. Those who have studied the mathematics of infinity, will know that there are more such paths than points on the number line.
With the pandemic’s horror now beginning to loosen its grip on all of us, I travelled back to Princeton last month to spend a few weeks with my family who still live there,
I came to reconnect with friends and family who I hadn’t seen in years, and also to search for the point of origin of my particular path. This is what I found.
Where it all began
My father, Lee Neuwirth, grew up in South Orange NJ, but after graduating high school in 1951, his brilliant mind gained him acceptance into Princeton where he became one of the handful of Jewish students that the University allowed to enroll each year.
Except for brief stretches spent in other academic settings, my dad never left the area, and 70 years after he arrived, he and my mother, who grew up in Bellville NJ still live in a modest 3- bedroom house directly across the street from the campus itself
My parents got married in 1955 just before my father graduated with a BSE in Chemical Engineering. In 1956 I was born, and for the first 10 months of my life I lived in Livingston NJ, a town just down the road from where all my grandparents lived. When my father was accepted to graduate school at Princeton, my parents bought a house on a country road a few miles outside of town and even though I have no memory of it, I celebrated my first and second birthdays there. We lived there until I turned 12 and then moved into town where my parents have lived ever since.
After graduating college my father decided that he didn’t want to be an engineer and so he went to Columbia where he studied “applied” math which he liked better. After two years of commuting to New York City, he decided that even applied math was too concrete for his tastes and returned to Princeton’s Graduate College just down the road from the undergraduate campus. There he studied topology – the branch of theoretical mathematics that analyzes the structure of multidimensional Space, the objects embedded in it and how each can be transformed while still maintaining its essential characteristics.
When my father attended this sanctuary of abstract thought, both as an undergraduate and graduate student, some of the immortals were still there. In lectures he attended were Einstein and Von Neumann, and he once attended a tea where Godel was present. Many others of almost equal luminosity were his teachers and future colleagues.
Shortly after getting his Ph.D. my father obtained a post-doctoral research fellowship at UC Berkeley where we lived for a year. He quickly became friends with the mathematicians there who were exploring other regions of the mathematical world. As was the case while he was in grad school, my father spent much of his time around other mathematicians — some of whom were as brilliant as the professors who had taught him at Princeton.
I was 4 years old, and my memories of that time included being entertained by these unusual geniuses.
Among my favorites was Moe Hirsch who was an accomplished musician, playing multiple instruments both at clubs in town as well at the house parties he and his wife Charity hosted. The friendship that Moe and my father formed that year has lasted until today. Despite being a serious mathematician like my father and deeply respecting the ancient roots of modern thought, Moe was also open to the modern world and when I was 9 he gave me my first Beatles album as a birthday present (“Beatles 65”) – music that Moe recognized as being created by real artists and potentially timeless in its appeal. My father, on the other hand considered the Beatles and “Beatlemania” a transitory phenomenon that would be soon forgotten as kids lost interest and moved their attention to the “next big thing”.
Also at Berkeley at that time was Stephen Smale, now recognized as one of the great mathematicians of our time who was an expert in dynamical systems – the general field mathematics in which Chaos occurs — that phenomenon that generates the beautiful fractals we have all seen and has the property of being both deterministic and impossible to predict. For my money this revelation is almost as philosophically important as Godel’s proof that some things are true but can never be proven.
My father focused on Knot Theory, and he became one of the recognized experts in that particular and relatively uncharted corner of the mathematical map.
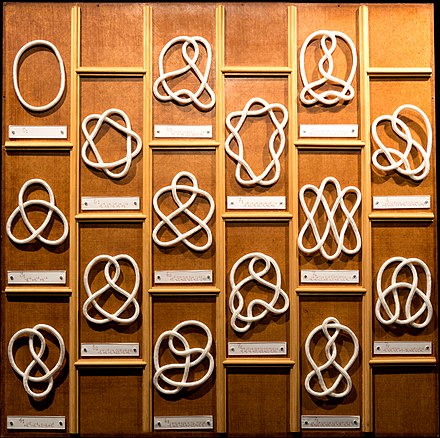Eventually my father’s work was featured on the cover of Scientific American and in 1965 Princeton University Press published his results in a real book (“Knot Groups”). When I expressed excitement about that, my dad told me that while several hundred copies were printed, he thought that there were no more than 12 people in the world who would be interested enough to read and fully understand what he had written.
His work was well respected and as a result, he became a member of a unique tribe of human beings for whom the material world is sometimes just an annoying distraction. They were a bizarre, but fascinating bunch and while they often ignored the “real” world, their impact on it was sometimes immense. Often, however, the connection to our daily lives is not so obvious and for many of these beautiful and abstract truths, the connection to our lives is yet to be found. For example, John Milnor, who my dad considered the best mathematician he ever knew is known for having discovered that 7-dimensional spheres are structurally different from each other, while spheres in lower dimensions (e.g , a circle which is a one dimensional sphere and the surface of a globe which is 2-dimensional ) are all structurally the same. Whether and how this incredible result will ever be manifested in what we see, touch and feel is an open question. Given the track record of how other great mathematical discoveries have been manifested in our technology I am not willing to bet against even Milnor’s findings being relevant to our material world.
Individually and in groups they explored the terrain of a vast (perhaps infinite) unseen wilderness. I learned early on to understand that when my dad was “doing mathematics” he was not to be disturbed as, in a very real sense, he was not in the same room. He would often come back from his mathematical journeys and try to tell me about the wonderous beauty he had seen, but his efforts were largely unsuccessful even though I picked up enough to make my math classes very easy to navigate – at least until they required hard work, something I avoided from a very early age
My parents now live in a small house adjacent to campus and count among their neighbors Simon Kochen, who, with the now deceased John Conway (another friend of my father’s) proved that if free will exists for us, then elementary particles have free will as well. A few doors down from Kochen was the home of Andy Wiles who proved Fermat’s Last Theorem – a mathematical result that had remained unproven for centuries until Wiles cracked the code and demonstrated that Fermat’s intuition was absolutely and demonstrably accurate.
For well over a hundred years Princeton has been home to great minds who looked inward and found great truths about the material world.
Einstein proved that nothing in the Universe, not even Space and Time, looks the same from all perspectives, Godel proved that no mathematical system is complete and knowable as there are statements that can be made in all branches of Mathematics that are true, but can never be proven.
John Von Neuman, considered by some to be the greatest mathematician of his time laid the groundwork for many of the most important fields of study of our time – including Quantum Mechanics, Game theory, and Computer Science. His calculations also significantly contributed to the building of the Atom Bomb.
My father’s expertise in his corner of the mathematical map eventually came to the attention of some of the higher-ups in the Defense Department in the Kennedy Administration, and when we returned from our year in Berkeley, the cryptologists that had been working in relative secrecy at the National Security Agency at Fort Meade reached out to my father and asked him whether he might be open to working on “some interesting problems that we can’t tell you about until you accept the job”
It was an offer my father was too intrigued to pass up and in 1961 he joined a select group of some of the best theoretical mathematicians of his generation to work with the most powerful computer in the world trying to break the secret codes of our enemies around the world.
They toiled in a non-descript brick building at the edge of the University campus, named after John Von Neuman. A painting of the great man hung in the lobby, the only part of the building I ever was ever allowed to enter. My father’s work was as secret as secret gets, with only those with “clearance” allowed to know what he did all day. As a result, neither he nor any of his colleagues were allowed to speak about their work to their families or any of their friends. Not surprisingly, most of my family’s social life centered around the families of the 60 or so staff who worked in Von Neumann Hall.
My father may have wanted to spend his life working in a University doing theoretical math and teaching students, but instead he chose to devote much of his gifts to helping his country, by learning how to read the secret messages of those who sought to harm the USA.
Many know the story of Alan Turing and the success that the British code breakers had in deciphering the German communications in World War II, but less well known is the fact that their American counterparts were hard at work and as successful at breaking the codes of the Japanese and the Russians during that same time. After the war, that group continued its work (as did the British), and in order to tap into the best mathematical minds in the country they established the Institute for Defense Analyses—Communications Research Division and leased a building on the Princeton University campus close to where the mathematicians they sought lived and worked.
In retrospect, it was obvious what they were doing there, but when my second grade teacher had a “show and tell” day where all of us were supposed to come in and share with the class what our fathers did for a living, I was the only one who had to say that I know where he works, but I am not allowed to know what he does. It made me feel very special, but it didn’t stop me from being extraordinarily curious and spending much of my childhood reading Sherlock Holmes and trying to find out the truth of things from the clues that are left behind.
For my father, the power and the importance of what Princeton had to offer him is summed up in a quote engraved on one of the extraordinary buildings scattered around the University. It speaks of learning about “unseen things that do not die.” The entire quote can be seen in the photo below.
The words that spoke to my father also resonate with me as well, even though I never saw it until my trip home this summer. To me it is a reminder not just to learn about these eternal truths that illuminate the deep structure of the Universe that we are a part of, but to also recognize that much of what moves the world forward is unseen and we would do well to keep that in mind any time we think we know what is happening now and what will happen next.
When I think about my point of origin, I realize how incredibly lucky I was to have the father I did and to grow up in Princeton. It keeps me curious, it helps me appreciate the wonder of what the human mind is capable of, and most of all it helps me realize that “not knowing” is not something to fear, but rather is something to be embraced as an essential part of the human condition.